Introduction

All objects have a certain amount of aerodynamic drag. In this article, I will be looking at how drag affects a vehicle's ability to accelerate, and the top speed. I will not be covering the actual study of automotive aerodynamics in detail.

Theory

Power

Power is defined as the rate of energy transfer, or the rate of work. A car's engine produces a certain amount of power, which can be used to exert a certain amount of force at a certain speed. I will be using horsepower as the unit of measure for power.

One horsepower is 33,000 foot-pound-force per minute, as defined by James Watt. The units for horsepower can be changed, as long as they remain the product of force and distance over time, and any necessary unit conversion constants are used. Below are the units for horsepower best suited for working with aerodynamics.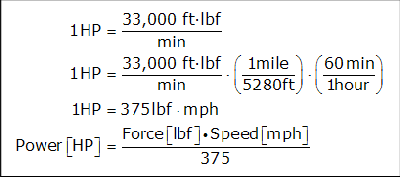

The definition of one horsepower, with units converted to show the force at a certain speed in miles per hour.

From the above equation, it can be seen that an engine producing one horsepower can exert 375lbf at 1mph, or 1lbf at 375mph, or any other equal product of force and speed. The formula can then be rearranged to obtain the force from the speed and power, as seen below.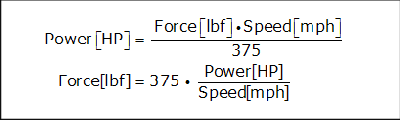

The force can be calculated when the power and speed are known.

Drag

The force of drag on an object varies with the following:

• The density of the fluid, ρ.
  The density varies with the temperature and pressure of the fluid ρ(T,p).

  The square of the velocity of the fluid, v.

  The drag coefficient of the object, Cd, a dimensionless parameter.

  The frontal area of the object, A.

The drag coefficient, Cd, is a dimensionless parameter that describes how much drag is caused by fluid flow on an object. A larger C_d value implies that the object has more drag.

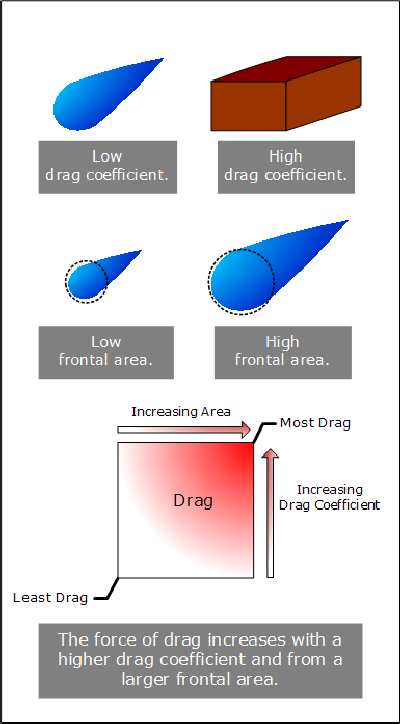
The drag properties of an object are dependent upon the shape and the size of the object.

The object's force of drag comes from its drag coefficient multiplied by the frontal area along with the velocity and density of the fluid, as seen below in the drag equation: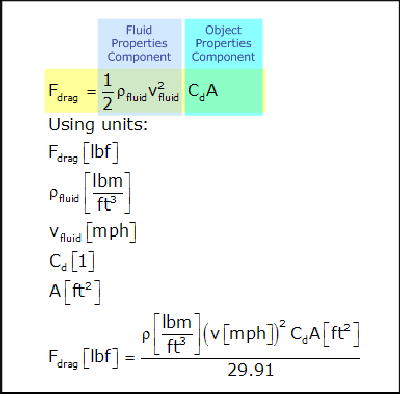

The drag equation, modified for use with units commonly used with cars.

The force of drag is present at speed, which means that drag is using power. At the same time, the engine is producing power at speed, which means it is exerting a force. The force that the car can use for acceleration is the force that is available after some has been used to press against the air. Because the speed of the air is the same as the speed of the car (assuming a calm day), the force of the engine and the force of the air can be compared directly.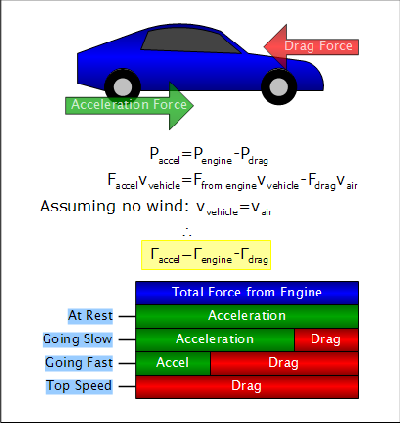

The engine exerts a force, while the aerodynamic drag opposes the engine's force. The remaining force from the engine is used for acceleration.

Acceleration and Top Speed

At very low speeds, the effects of aerodynamic drag are minimal. At the same time, the engine can exert a very large force at low speeds, meaning that the acceleration force will be high. At higher speeds, the drag builds up, and the engine's force decreases. Eventually, the drag force and engine force will become equal, leaving no force left for acceleration. It is at this speed that the car is said to have reached its drag-limited top speed.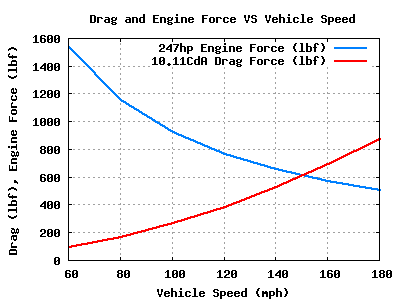

The above graph shows the force that the engine can exert, and the force due to aerodynamic drag. When the drag force becomes equal to the force the engine can exert, the car reaches its drag-limited top speed. In this case, the car's top speed is approximately 150mph.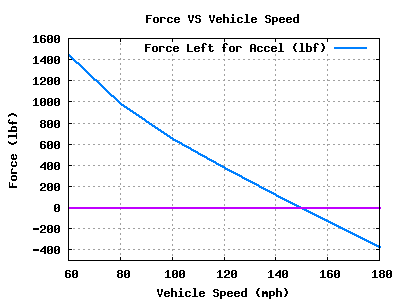

The above graph shows the force available for acceleration after drag has been added. When the force is zero, there is no longer any acceleration.

If the car's power and drag are known, the drag-limited top speed can be calculated by combining the drag equation with the horsepower equation, as seen below: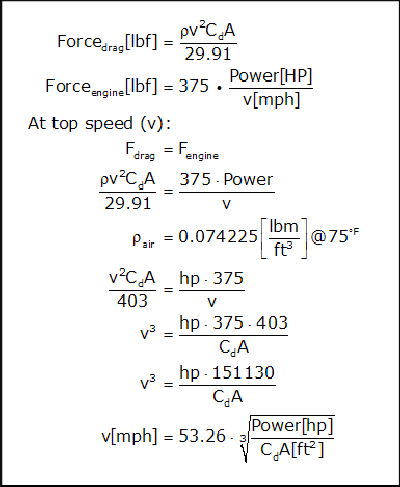

The top speed can be determined from the horsepower and drag of the vehicle by setting the force of drag equal to the force of the engine.

Comparison

To help show the effects of drag on a vehicle, I will do a simple comparison. This time, I'll be using vehicles right from my family's fleet: my Dad's '87 Supra and my '82 Caprice.

The contenders: A 1987 Toyota Supra Turbo with a slippery body against a 1982 Chevy Caprice with a boxy body.

For this comparison, I will be adding some horsepower to the Caprice. It currently has 180hp, but I'm going to give it 247hp for this experiment, to give it the same power-to-weight ratio as the Supra. This will be done to remove the effects of the weight difference between the cars. I will also make the assumption that the cars can lay down their peak power at any speed, which will remove the effects of driveline gearing. Finally, acceleration forces will not be shown, because all of the values would be nonsense with these assumptions applied.

Below is a table showing the relevant values for the cars.

Using the values from the above table and the equations presented earlier, the performance of the cars can be determined at any vehicle speed. Below is a table comparing the two cars side by side. The acceleration ability is related to the ratio of the horsepower available for acceleration and the weight of the vehicle.

It can be seen in the table above that both cars have the same acceleration at low speeds, but as the speed is increased and the drag builds, the less aerodynamic car begins to fall behind. Even though the difference in top speed is a mere 16mph, the Caprice was losing the race at around 100mph, which is well below either car's top speed.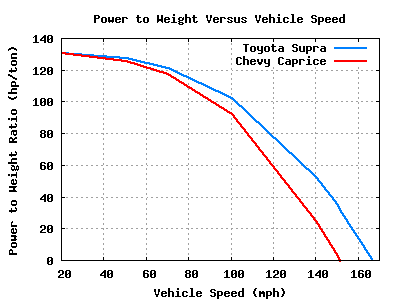

Graphical comparison of the Supra and Caprice.

It would take approximately 332hp to get the Caprice to reach the Supra's top speed of 166mph. Of course, if the Caprice had that much power, it would blow away the Supra at lower speeds. This shows that the Caprice wastes a lot of power on fighting the air.

Practicality

Gearing

So far in this article, I have assumed that a car can put out its peak power no matter what speed it is travelling. In real life, this is not the case because a car's engine does not always operate at peak power. Therefore, a car's top speed may be much lower than expected. Generally, a car with many transmission gears to choose from will be able to approach its theoretical top speed, while a car with very few gears could be way off, because the engine will be nowhere near its power peak.

Some cars, especially very old performance cars, do not hit their aerodynamic top speed, but rather run out of gears. If a car hits its redline in top gear before reaching its drag-limited top speed, the car is said to have a gear-limited top speed. This is not very common in modern cars, which have many transmission gears, and axle gearing configured for fuel economy.

A car will always have a top speed equal to its gear-limited top speed, or its drag-limited top speed, whichever is lower.

Gas Mileage

A car needs a certain amount of horsepower to push against the air at speed. Power in a gas engine is produced by burning fuel, so a vehicle with more drag will need to burn more fuel to move at the same speed as a more aerodynamic vehicle.

The aerodynamic drag varies with the square of the vehicle speed, so if the speed of the vehicle is doubled, the drag quadruples. From this, it follows that the drag at 70mph is twice the drag at 50mph.

Having said that, a car will not necessarily get better gas mileage at 50mph than 70mph. Gas mileage depends on many other things which are beyond the scope of this article.

Braking

Aerodynamic drag can be used to help stop the vehicle. If someone were to drive their car very fast and then put the clutch in suddenly, the car would begin to decelerate because the aerodynamic drag force opposes the car's motion. Combining the drag force with the braking force (and forces from friction) allows the car to decelerate at a very high rate when traveling at high speeds. This is why some sports cars have flaps that come up under high-speed braking. The flaps can also aid in stability under braking by helping to press the rear wheels down onto the pavement.

Other Factors

There are many things that will prevent a car from reaching it's theoretical top speed. Below is an incomplete list.

1. Friction between the road and the tires.
2. Power used to build momentum in the wheels and other moving parts.
3. Instability at high speeds.
4. Lack of a suitable facility (hills, etc).
5. Wind.
6. The law.

Conclusion

Aerodynamic drag is a significant factor in a car's ability to accelerate. At very high speeds, the ratio of the engine's power output to the body's drag becomes more important than the power-to-weight ratio, which is important at lower speeds where drag is not significant. Assuming that the car has suitable gearing, the only way to increase the top speed would be to improve the aerodynamics, or add power. In ideal conditions, the weight of a vehicle has almost no effect on top speed, only the time it takes to get there.

See Also

• Horsepower and Torque